Steam Drum Level
Steam drum level measurement with a differential pressure transmitter
can be a tricky business when the pressure is higher than for "low"
pressure steam. What happens is that as the temperature rises, the
density of water drops while at the same time that of steam rises. To
compound the problem, the wet leg temperature is not well defined and
its density is a third variable.
A technical way around the wet leg problem is to use the following
level capture apparatus.
.
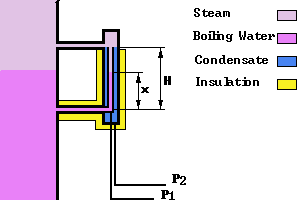The constant condensation in the top connection maintains a constant
influx of hot water at equilibrium with the steam. This maintains the
heat and ensures both wet leg and measurement sections are at the same
temperature (that of the water in the steam drum), below the apparatus,
the two impulse lines are in close contact and therefore at the same
temperature. Whatever the density of the water is, it is the same in
both legs and cancels out in the differential measurement.
Within the apparatus itself, the dilemma remains. The simplest way is
to use a correlation to provide the steam and the water densities as a
function of the absolute pressure in the boiler.
Looking at figure 1, we see that the differential pressure,
P2 - P1 is:
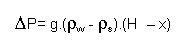
where g is the gravitational acceleration constant:
9.807 m/s2, and
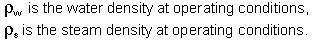
Furthermore we assume the transmitter is calibrated with cold water
which has a density of:
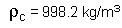
We note that when the level is 100%, the differential pressure is 0,
regardless of whether the water is hot or cold. When the level is 0%,
the differential pressure is maximum although this maximum value depends
on the pressure in the steam drum.
Now, assuming the transmitter has a reversed output, Lraw,
calibrated with cold water, so that when the level increases so does
the analog signal, then it is possible to express the level, L, in %
as a function of the densities of the water (both hot and cold) and of
the steam:
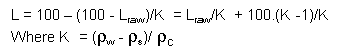
To do so I have calculated three fourth degree polynomials which
predict the density of steam, water, and the correction term K as a
function of pressure. Those polynomials have been derived using the
ASME steam tables and using least square regression to obtain the best
fit.
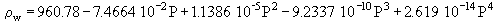
The densities are in kg/m3 and the pressure is in kPa
absolute.
For the steam density the relative error is less than +/-1% for all
pressures between 500 and 15000 kPa(abs). The relative error becomes
larger at low pressures being 6% at 100 kPa(abs)
For the water density and for K the relative error is +/-1% or less
from 0 kPa(abs) to 15000kPa(abs)
This relatively simple formula will correct the dP measurement and
indicate the correct level from stone cold to over 340° C.
Do not hesitate to email me
for any question about this set-up!
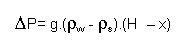
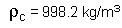
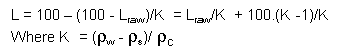
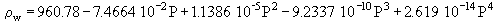
Return